Cho \(x, y \in \mathbb{R} \text { thỏa } \text { mãn } x+y \neq-1\) và \(x^{2}+y^{2}+x y=x+y+1\). Gọi M , m lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của biểu thức \(P=\frac{x y}{x+y+1}\). Tính M+m.
Suy nghĩ và trả lời câu hỏi trước khi xem đáp án
Lời giải:
Báo sai\(\text { Với điều kiện } x+y \neq-1 ; x^{2}+y^{2}+x y=x+y+1 \text { ta có } P=\frac{x y}{x^{2}+y^{2}+x y} \text { . }\)
\(\text { Nếu } y=0 \text { thì }\left\{\begin{array}{l} x \neq-1 \\ x^{2}-x-1=0 \end{array} \Leftrightarrow x=\frac{1 \pm \sqrt{5}}{2} \text { . Khi đó } P=0\right. \text { . }\)
\(\text { Nếu } y \neq 0 \text { thì } P=\frac{\frac{x}{y}}{\left(\frac{x}{y}\right)^{2}+\frac{x}{y}+1} \text { . Đặt } t=\frac{x}{y} \text { . Ta có } P=\frac{t}{t^{2}+t+1}, t \in \mathbb{R} \text { . }\)
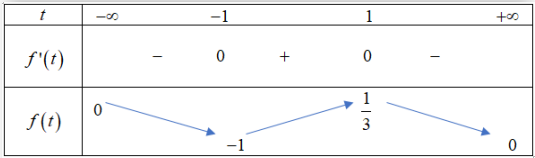
\(\text { Xét } f(t)=\frac{t}{t^{2}+t+1}, t \in \mathbb{R} \cdot f^{\prime}(t)=\frac{-t^{2}+1}{\left(t^{2}+t+1\right)^{2}} ; f^{\prime}(t)=0 \Leftrightarrow t=\pm 1\)
Từ bẳng biến thiên:
\(M=\frac{1}{3} \text { tại }\left\{\begin{array} { l } { \frac { x } { y } = 1 } \\ { x ^ { 2 } + y ^ { 2 } + x y = x + y + 1 } \end{array} \Leftrightarrow \left\{\begin{array}{l} x=y \\ 3 x^{2}-2 x-1=0 \end{array}\right.\right.\)
\(\Leftrightarrow \left\{ {\begin{array}{*{20}{l}} {x = y}\\ {\left[ {\begin{array}{*{20}{l}} \begin{array}{l} x = 1\\ x = - \frac{1}{3} \end{array} \end{array}} \right.} \end{array}} \right.\)\(\Leftrightarrow\left[\begin{array}{l} x=y=1 \\ x=y=-\frac{1}{3} \end{array}\right.\)
\(m=-1 \text { tại }\left\{\begin{array} { l } { \frac { x } { y } = - 1 } \\ { x ^ { 2 } + y ^ { 2 } + x y = x + y + 1 } \end{array} \Leftrightarrow \left\{\begin{array}{l} x=-y \\ x^{2}-1=0 \end{array}\right.\right.\)\(\Leftrightarrow\left\{\begin{array}{l} x=-y \\ {\left[\begin{array}{l} x=1 \\ x=-1 \end{array}\right.} \end{array}\right.\)
\(\Leftrightarrow\left[\begin{array}{l} \left\{\begin{array}{l} x=1 \\ y=-1 \end{array}\right. \\ \left\{\begin{array}{l} x=-1 \\ y=1 \end{array}\right. \end{array}\right.\)
\(\text { Vậy } M+m=-\frac{2}{3} \text { . }\)
.png)
.jpg.png)